Super-resolution for computed tomography based on discrete tomography
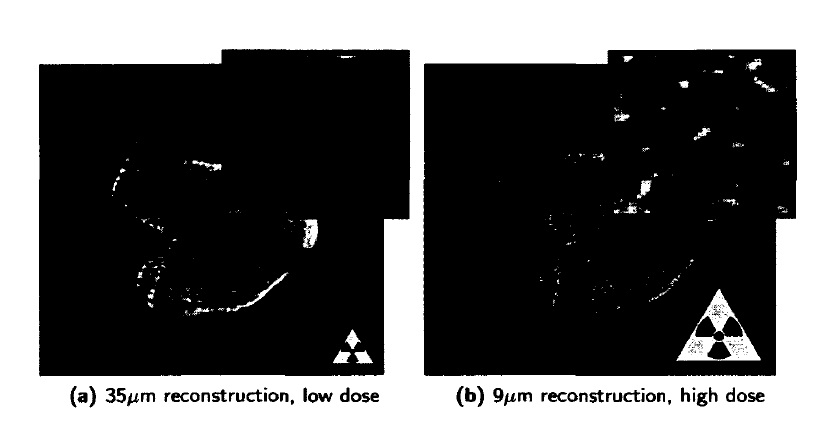
Figure 1: (a) Reconstruction of a polyurethane foam, scanned in a SkyScan 1172 /zCT
scanner at a pixel resolution of 17/y.m. (b) Otsu’s segmentation of the reconstruction. Many
cell walls remain undetected in the segmentation while other structures are overestimated
In X-ray computed tomography, images are typically reconstructed on a voxel grid. Given that each voxel is represented by a constant grey level, it is typically assumed that the material within such a voxel is homogeneous. It is clear, however, that a voxel representation cannot properly represent structures that have a varying density within a voxel. Thus, each voxel in the images could contain more than one material or tissue type. This phenomenon is referred to as the partial volume effect (PVE). PVEs cause object boundaries to be smeared out across the boundary voxels. Also, if a feature of the scanned object is small relative to the nominal voxel size, PVEs reduce the contrast between the structure of interest and its background signal. Consequently, it is difficult to achieve the intrinsic resolution of the detector. Fig. 1 a shows an FBP reconstruction of a polyurethane foam for which the widths of the edges of the pores are comparable to the detector size. A globally thresholded segmentation of Fig.1a, created with the commonly used clustering method of Otsu, is shown in Fig. 1b. Clearly, many thin structures remain undetected, whereas the thickness for some larger structures is overestimated.To reduce PVEs — and hence to obtain sufficient contrast a high resolution scan can be acquired. This, however, requires a high radiation dose and a longscanning time . In Fig.2, an FBP reconstruction with a spatial resolution of 35/xm is shown of a rat femur along with an FBP reconstruction with a spatial
super-resolution scheme is based on the Discrete Algebraic Reconstruction Technique (DART), as presented in Chapter 4. It it shown that by upsampling the reconstruction grid and incorporating available prior knowledge to compensate for the lack of high resolution projection data, the proposed approach effectively increases the spatial resolution of the tomographic reconstructions

Figure.2: FBP reconstructions of the epiphyseal plate of a rat femur taken at two different resolutions in a SkyScan 1172 f/CT scanner. The high dose reconstruction (b) is clearly much easier to segment. Note that as both slices were taken from different scans, the object was slightly displaced between the acquisition of both datasets. Even though image registration was performed, there is still a residual difference
resolution of 9/xm of the same femur. It is clear that the contrast in Fig. 2b is significantly better than that in Fig. 2a. Fig.2b is therefore much better suited for accurate segmentation and estimation of the morphometric bone parameters [2]-The conventional approach to reduce PVEs without increasing the X-ray dose is to upsample the reconstruction voxel grid, allowing for a more accurate representation and potentially improving the overall visualization of small structures. This upsampling is also known as super-resolution. Conventional reconstruction upsampling typically results in a limited data reconstruction problem: the number of equations (measured projection data) remains the same while the number of unknowns (reconstruction voxels) increases significantly. This problem can be overcome by combining the information from multiple low resolution CT images into a high resolution CT image, but this would again result in an increased scan time and radiation dose. In this chapter, a super-resolution approach for CT is proposed that effectively solves the limited data problem by incorporating some form of prior knowledge about the unknown object. In CT, such prior knowledge comes in many forms, e.g. sparsity of the reconstructed image or its gradient . Here, the novel super-resolution scheme is based on the Discrete Algebraic Reconstruction Technique (DART). It it shown that by upsampling the reconstruction grid and incorporating available prior knowledge to compensate for the lack of high resolution projection data, the proposed approach effectively increases the spatial resolution of the tomographic reconstructions